
where students teach themselves
A comprehensive geometry curriculum
Geometry for School and Home
Activity 3.8
Assignment 3.9
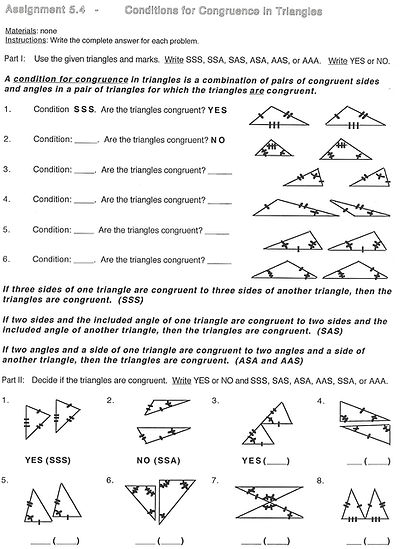
Assignment 5.4
Conditions for Congruence in Triangles
Objectives
Identify conditions that justify triangle congruence.
Given pairs of triangles with pairs of congruent sides and angles, identify the triangles that are congruent and the justifying condition.
Next
Previous
About Geometry Worksheet Assignment 5.4:
Assignment 5.4 is an introduction to conditions for congruence in pairs of triangles.
Prerequisite Skills: Identify congruent pairs of sides and angles in pairs of triangles using the letters S and A (Assignment 5.2). Identify legs and hypotenuses in right triangles (Assignment 3.13). Identify included sides and included angles in triangles (Assignment 5.3).
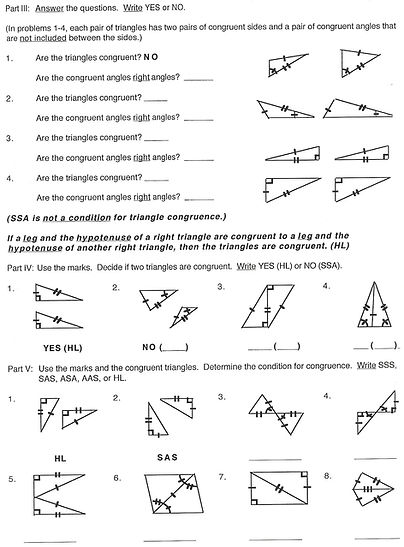
Definition: A condition for congruence in triangles is a combination of pairs of congruent sides and angles in a pair of triangles for which the triangles are
congruent.
Postulates: If three sides of one triangle are congruent to three sides of another triangle, then the triangles are congruent (SSS).
If two sides and the included angle of one triangle are congruent to two sides and the included angle of another triangle, then the triangles are congruent (SAS).
If two angles and a side of one triangle are congruent to two angles and a side of another triangle, then the triangles are congruent (ASA and AAS).
If a leg and the hypotenuse of a right triangle are congruent to a leg and the hypotenuse of another right triangle, then the triangles are congruent (HL).